|
|
SCIENTIFIC BACKGROUND
The formulas taken as the starting points for our project are nonlinear differential equations. Some of them exhibit particularly curious solutions, which have come to be known as "chaotic" or "strange" attractors. Although these solutions are fully determined by the underlying equations, they nonetheless contain unpredictable, non-periodic behaviors.
In the late 19th century, French mathematician Henri Poincaré conducted foundational work on differential equations which suggested the existence of non-periodic solutions. But chaotic attractors only became popular in the 1960s. The best known chaotic attractor was found by accident in 1963, when meteorologist Edward Lorenz numerically approximated the solution for a nonlinear equation which described weather phenomena (the Lorenz attractor). Many people know of the Lorenz attractor (often together with the notion of the butterfly effect) as being the first of its kind. But it was in fact the Japanese scientist Yoshisuke Ueda who first encountered non-periodic behavior (also accidentally) during his analog computer experiments in 1961. The attractor he found looked like a shattered egg, and was later named "Japanese attractor" by the French mathematician Davide Ruelle.
In the 1970s, Otto Rössler purposely designed nonlinear equations with chaotic behaviors. His first and widely investigated result is named after him: the Rössler attractor. Later, many different equations were found all over the world. In the 1990s, for instance, J. C. Sprott developed a program which systematically searches a parameter space in order to find all the possible simple prototypes of chaotic attractors in 3 dimensions.
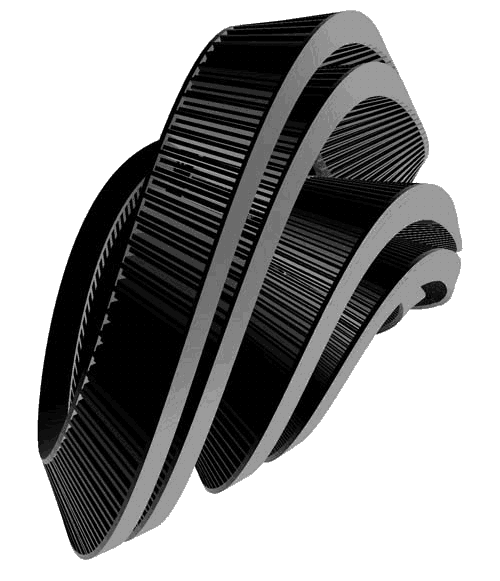
Since research into nonlinear systems with chaotic solutions often relies on numerical approximations, the visualization of results is of particular importance. In effect, the image is essential in effectively communicating the results. As such, hand drawings were often included for the demonstration of topological properties in early publications about chaotic attractors. Another common technique was the production of stereoscopic plots. Since the 1980s, in the field of nonlinear dynamics in particular, computers and computer graphics have been essential tools in finding, investigating and communicating results about strange attractors. But computer images remain something of a compromise, particularly for 3D nonlinear dynamical systems, since the solution is necessarily a three-dimensional trajectory represented on a two-dimensional screen. Our project, _zur form, aims to bring these new virtual images back to 3D reality, and situates them to this end in the established tradition of 3D mathematical objects.
For the installation, we have chosen to produce the Lorenz and the Rössler attractors, as well as three examples from Sprott's prototypes, all in 3D forms. Chaotic attractors are usually depicted as a single trajectory in one long thin line. In our models, we represent this line as a ribbon which indicates the principle directions of stability. These ribbons either make simple loops or wind like Möbius strips and allow for a visual interpretation of their curious topological structures.
Edward N. Lorenz: Deterministic Nonperiodic Flow.
In: Journal of the Atmospheric Sciences, Vol. 20, No. 2, 130-141, 1963
O.E. Rössler: An Equation for Continuous Chaos
Physics Letters Vol. 57A no 5, pp 397-398, 1976.
J.C. Sprott: Some simple chaotic flows
Physical Review E Vol. 50 no 2, R647-R650 1994
|
| info AT zurform.org |
top » |
|
|